Quiz de prérentrée

Primary tabs

Question 1

Quel est le domaine de définition dans $\mathbb{R}$ de la fonction suivante : $\dfrac{x-1}{e^x-2}$

Catégorie:

Votre réponseChoixCommentaireBonne réponse
Selected
$\mathbb{R} \setminus \{ \ln(2) \} $
Should have chosen
$\mathbb{R} \setminus \{ \ln(2) ; 1 \} $
Should not have chosen
$\mathbb{R} \setminus \{ 1 \} $
Should not have chosen
$\mathbb{R} $
Should not have chosen
Question 2

Quel est le domaine de définition dans $\mathbb{R}$ de la fonction suivante : $\dfrac{\sqrt{x-1}}{x-2}$

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$\lbrack 1 ; +\infty \lbrack$
Should not have chosen
Selected
$\lbrack 1 ; 2 \lbrack \; \cup \; \rbrack 2 ; +\infty \lbrack$
Should have chosen
$\mathbb{R} \setminus \{ 1 ; 2 \} $
Should not have chosen
$\rbrack -\infty ; -1 \lbrack \; \cup \; \rbrack 2 ; +\infty \lbrack$
Should not have chosen
Question 3

À quel système correspond la région blanche du graphique ?

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$\displaystyle{(S)\begin{cases} 2x+y & < 5\\ x-2y & < 8 \end{cases}}$
Should not have chosen
$\displaystyle{(S)\begin{cases} 2x+y & < 5\\ x-2y & > 8 \end{cases}}$
Should have chosen
$\displaystyle{(S)\begin{cases} 2x+y & > 5\\ x-2y & < 8 \end{cases}}$
Should not have chosen
Selected
$\displaystyle{(S)\begin{cases} 2x+y & > 5\\ x-2y & > 8 \end{cases}}$
C'est la région bleue. Il suffit de tester le point $(x=5 ; y=-2)$.
Should not have chosen
Question 4

Considérons le système suivant :
$\displaystyle (S)\begin{cases} 2x+y & = 10 \\ 3x-y & = 5 \end{cases}$
Quelle est la valeur de la solution $x$ ?

Catégorie:

Votre réponseCommentaireBonne réponse
2,53
Question 5

La fonction $x \mapsto x\sqrt{x}$ est dérivable en $x=0$.

Catégorie:

Votre réponseChoixCommentaireBonne réponse
Vrai
Should have chosen
Faux
Revenir à la définition de la dérivée et calculer la limite en $x=0$  de $\lim\limits_{h\to 0 \\ h>0} \frac{(x+h)\sqrt{x+h}}{h}$.
Question 6

La fonction $x \mapsto x\sqrt{x}$ est dérivable en $x=0$.

Catégorie:

Votre réponseChoixCommentaireBonne réponse
Vrai
Faux
Should have chosen
Revenir à la définition de la dérivée et calculer la limite en $x=0$  de $\lim\limits_{\substack{h\to 0 \\ h>0}} \frac{(x+h)\sqrt{x+h}}{h}$.
Question 7

Soit $f$ une fonction numérique définie sur l'intervalle $[-1;3]$ et $a$ un réel de cet intervalle.
Si $f$ est dérivable en $a$ elle est continue en $a$.

Catégorie:

Votre réponseChoixCommentaireBonne réponse
Selected
Vrai
Should have chosen
Faux

C'est un théorème du cours.

Question 8

Soit $f$ une fonction numérique et $\mathcal{C}_f$ sa courbe représentative dans le plan muni du repère $(O ; \vec{i} ; \vec{j} )$.
Si $f(-1)=0$ et si $f'(-1)=3$ alors la tangente à $\mathcal{C}_f$ au point d'abscisse $-1$ a pour équation $y=3x$.

Catégorie:

Votre réponseChoixCommentaireBonne réponse
Vrai
Selected
Faux
Should have chosen
L'équation d'une tangente au point $A(x_a ; y_a)$ doit impérativement passer par le point $A$. Vérifier si c'est le cas ici.
Question 9

Soit $f$ une fonction numérique et $\mathcal{C}_f$ sa courbe représentative dans le plan muni du repère $(O ; \vec{i} ; \vec{j} )$.
Si la tangente à $\mathcal{C}_f$ au point $B(1 ; 5 )$ est parallèle à la droite d'équation $y=2x + 1$ alors $f'(1)=2$.

Catégorie:

Votre réponseChoixCommentaireBonne réponse
Vrai
Should have chosen
Faux
La tangente en $B( 1 ; 5 )$ parallèle à $y=2x + 1$ permet d'obtenir son  coefficient directeur. Le coefficient directeur permet de déduire le nombre dérivé $f'(1)$.
Question 10

Soit $f$ une fonction dérivable sur $\mathbb{R}$. La courbe de sa dérivée est donnée ci-dessous.
$f$ admet-elle un maximum en $x=2$ ?

Catégorie:

Votre réponseChoixCommentaireBonne réponse
Vrai
Faux
Should have chosen
C'est le maximum de $f'$, pas de $f$.
Question 11

Soit $f$ la fonction définie sur $\mathbb{R}$ par: $\displaystyle f(x)=(x+1)e^{2x}$.
L'équation $f(x)=1$   admet dans $\mathbb{R}$ :

Catégorie:

Votre réponseChoixCommentaireBonne réponse

deux solutions.

Should not have chosen

une unique solution.

Should have chosen
aucune solution.
Should not have chosen
Question 12

$\displaystyle \lim\limits_{x \to +\infty} \frac{2e^x+1}{e^x+2}$ est égale à :

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$+\infty$
Should not have chosen
$2$
Should have chosen
$1$
Should not have chosen
$\displaystyle -\frac{1}{2} $
Should not have chosen
Question 13

La fonction $f \colon x \mapsto e^{-x}$ est :

Catégorie:

Votre réponseChoixCommentaireBonne réponse
croissante sur $\mathbb{R}$.
Should not have chosen
décroissante sur $\mathbb{R}$.
Should have chosen
négative sur $\mathbb{R}$.
Should not have chosen
Question 14

L'expression $e^x(2e^{-x}-1)$ est égale à :

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$2e^{-x^2}-e^x$
Should not have chosen
$-2(e^x)^2-e^x$
Should not have chosen
$2-e^x$
Should have chosen
Question 15

L'inégalité $\ln (x-1) < 1$ est vérifiée pour :

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$x>1$
Should not have chosen
$x<1+e$
Should not have chosen
$1 < x < 1+e$
Should have chosen
Question 16

L'égalité $\displaystyle e^{\ln x}=x$ est vrai pour tout $x$ appartenant à :

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$\mathbb{R}$
Should not have chosen
$\left]0;+\infty\right[$
Should have chosen
$\left[0;+\infty\right[$
Should not have chosen
Question 17

L'équation $e^x=-2$ a pour solution :

Catégorie:

Votre réponseChoixCommentaireBonne réponse

aucune

Should have chosen
$\ln (-2)$
Should not have chosen
$\ln(2)$
Should not have chosen
Question 18

Soit $f$ la fonction définie par $f(x)=\ln\left(x^2\right)$.
L'ensemble de définition de $f$ est :

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$\mathbb{R}^*$
Should have chosen
$]0;+\infty [$
Should not have chosen
$\mathbb{R}$
Should not have chosen
Question 19

Voici la courbe des fréquences cumulées croissantes du nombre d'enfants moyen par famille en France en 2007.

Parmi les 4 affirmations suivantes, laquelle est correcte ?

Catégorie:

Votre réponseChoixCommentaireBonne réponse
22 % des familles ont un enfant unique.
Should have chosen
70 % des familles ont au moins 1 enfant.
Should not have chosen
90 % des familles ont au moins 2 enfants.
Should not have chosen
3 % des familles ont au plus 3 enfants.
Should not have chosen
Pour trouver la fréquence des familles ayant un seul enfant, on fait le calcul $0,7 - 0,48 = 0,22$.
Question 20

Ce diagramme représente la répartition du nombre de buts marqués par match pour une équipe de football tout au long du championnat.

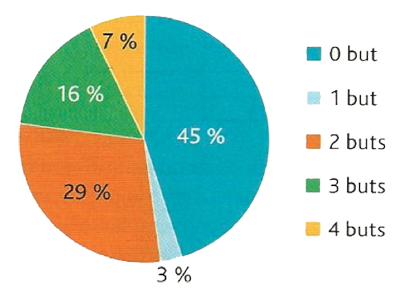
Le nombre moyen de buts marqués par match au cours du championnat est :

Catégorie:

Votre réponseChoixCommentaireBonne réponse
$\overline{x} = 2$
Should not have chosen

On ne peut pas savoir.

Should not have chosen
$\overline{x} = 0,5$
Should not have chosen
$\overline{x} = 1,37$
Should have chosen
On obtient ce résultat en faisant :
$\overline{x} = 0×0,45+1 \times 0,03+2 \times 0,29+3 \times 0,16+4 \times 0,07=1,37$